Patterns of diversity across space
Zooming out on Earth
Zooming out on Earth
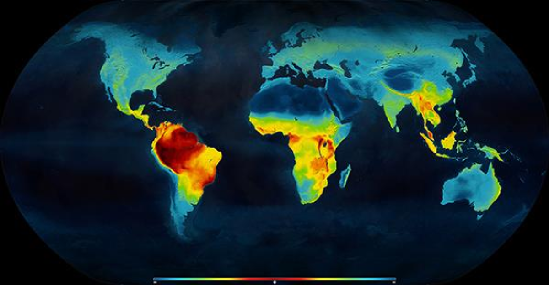
Diversity of vertebrate species, from Mannion et al. 2014
Zooming out on Earth

Diversity of amphibian species, from Anton-Pardo, 2019
Zooming out on Earth

Diversity of mammal species, from Davies et al. 2008
Zooming out on Earth
Diversity of Streptomyces bacteria, from Andam et al. 2016
Zooming out on Earth
Plotting the relationship between diversity and latitude
Zooming out on Earth
The latitudinal diversity gradient seems ubiquitous
Why does it exist?

Six potential hypotheses
- Time: Organisms have been able to evolve in the tropics for longer times than in temperate regions (e.g. due to polar glaciations)
- Environmental heterogeneity: There is more “complexity” in tropical environments, enabling more species to persist
- Biotic competition: Stronger competition between species in tropics leads to narrower niches
- Predation: Stronger predation in the tropics keeps down “dominant” species, enabling more to persist
- Climatic stability: More stable tropical climates promotes speciation(?)
- Productivity: Greater productivity of tropical climates allows more species to be “packed in”
Contemporary explanations
Emphasis on rates of speciation and diversification
Explaining patterns of diversity
S=speciation−extinction
S=speciation−extinction+immigration
But, we still lack clear answers

Diversity of marine fish species, from Rabosky et al. 2018
But, we still lack clear answers

Patterns of diversity across space
Mid-semester survey results
- Positive attitudes towards reflections & activities
- Some uncertainty about semester project – to be clarified in coming week
- Desire for more activities in class to solidify understanding
Overview
How is biodiversity structured at large and small spatial scales?
How can we quantify biodiversity?

Diversity of vertebrate species, from Mannion et al. 2014
- Negative slope between diversity and absolute latitude
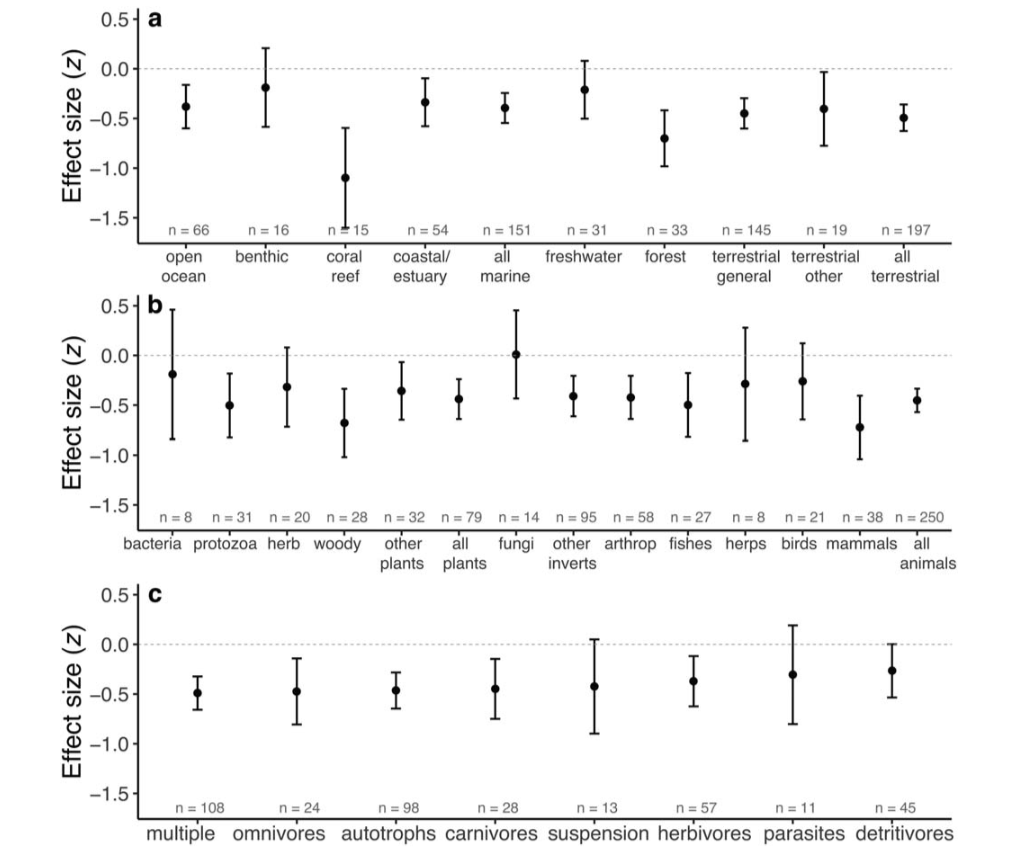
 Meta-analysis of diversity, from Kinlock et al. 2018
Meta-analysis of diversity, from Kinlock et al. 2018
But, nature holds surprises

Diversity of Saccharomycotina yeasts, from David et al. 2024
But, nature holds surprises

Diversity and speciation rates of marine fish species, from Rabosky et al. 2018
To accurately define patterns of diversity, we need accurate measures of diversity.
Note: there are many dimensions to diversity that we won’t cover in depth.
- Phylogenetic diversity
- Two communities with 10 species each – in the first, all species from the same genus; in the second, 8 genera represented
- Functional diversity
- Two communities with 10 species each – in the first, each of the 10 is a herbivore; in the second, an even mix of herbivores, omnivores, carnivores
To accurately define patterns of diversity, we need accurate measures of diversity.
Today, we will focus on measuring “evenness” as a dimension of quantifying diversity
- Using local birds as a case study












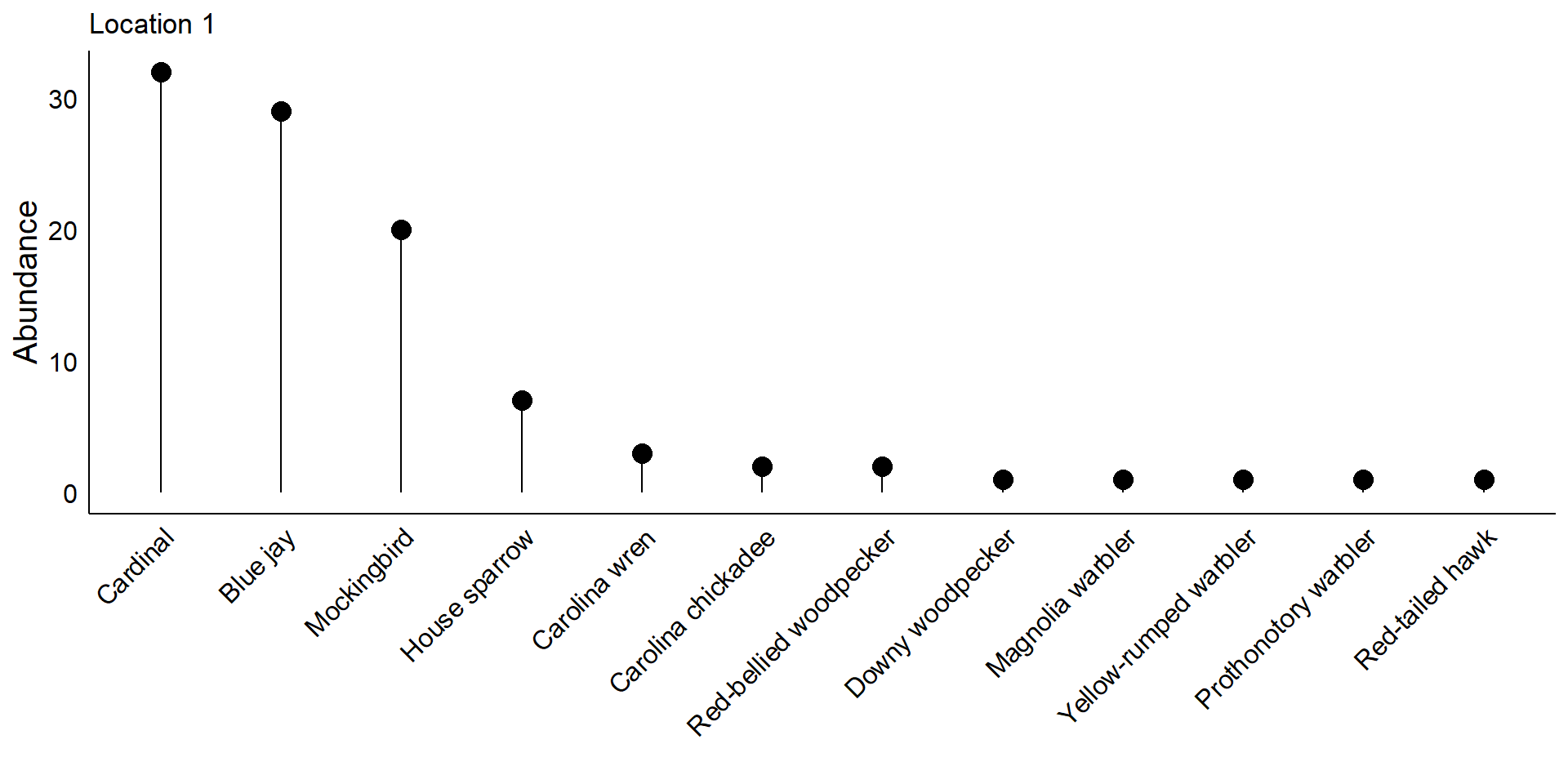
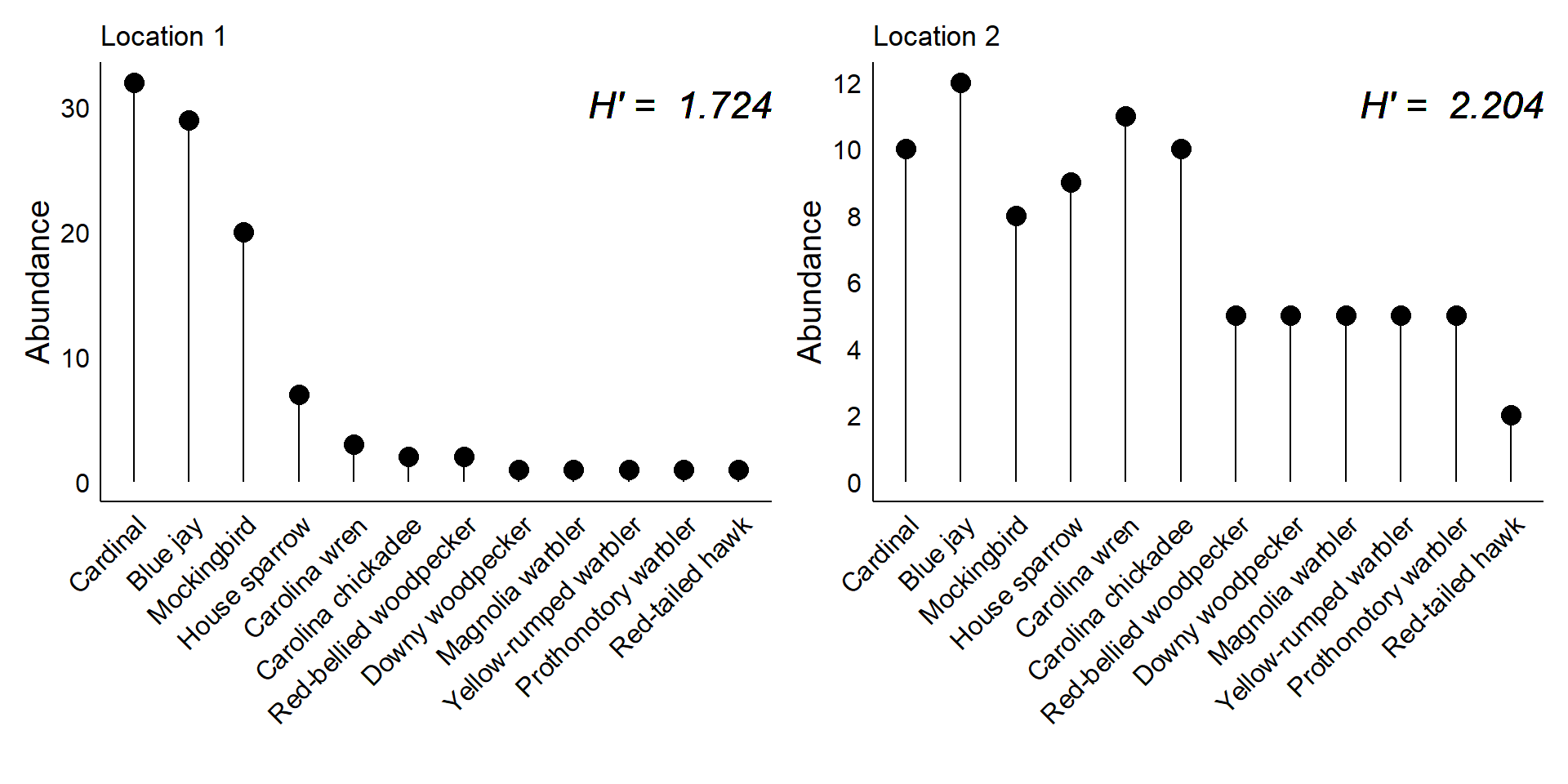
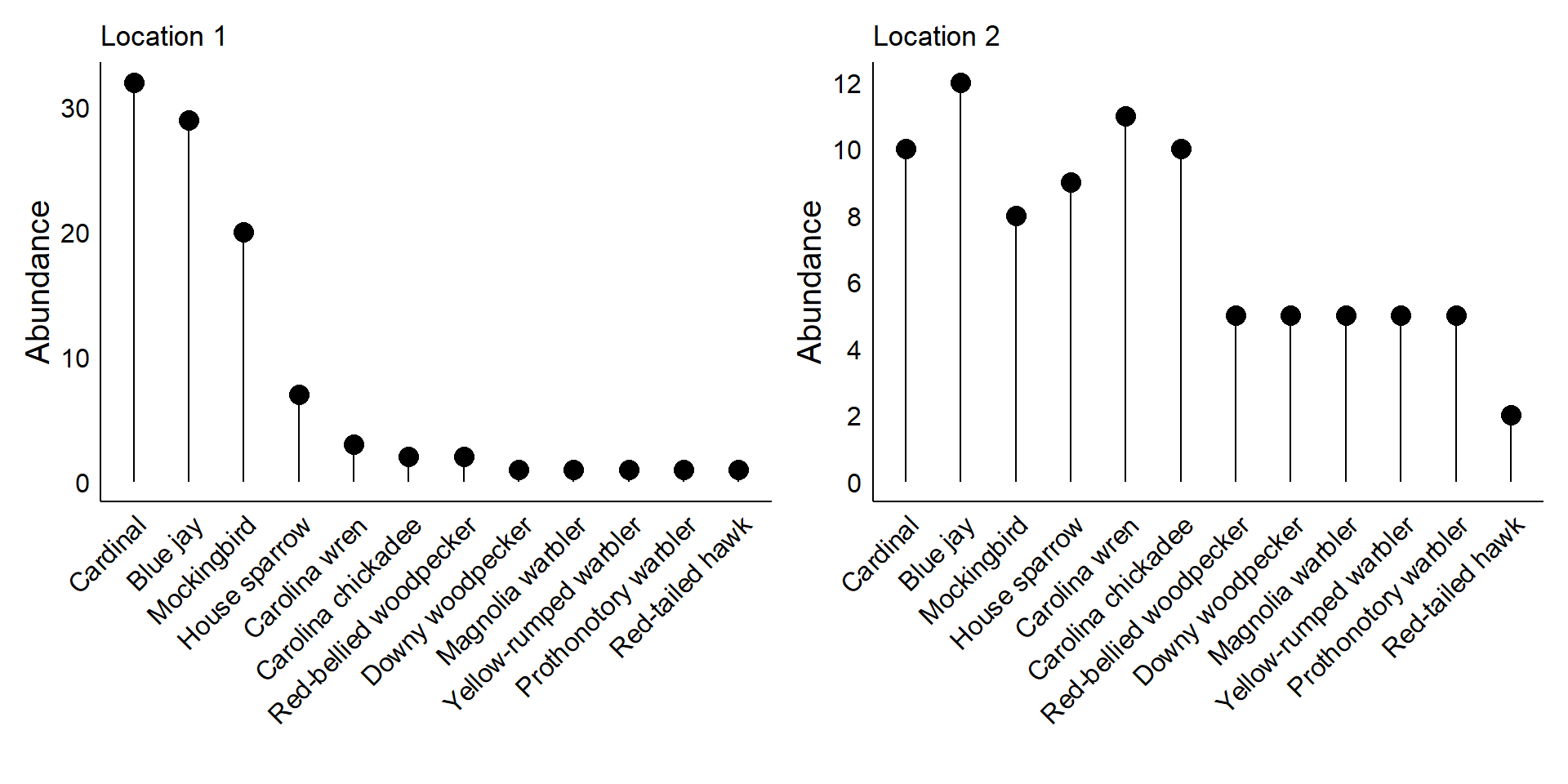
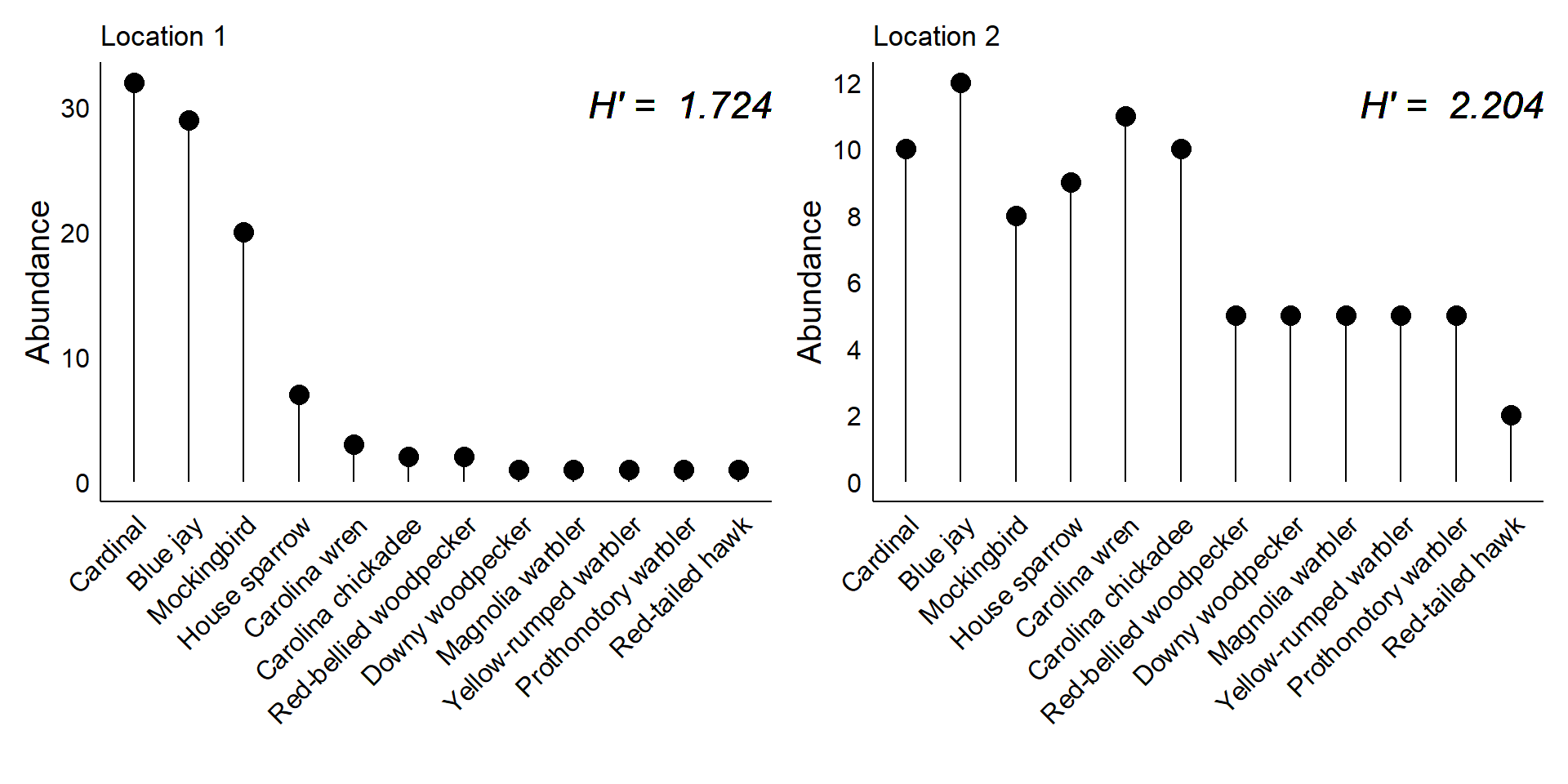
Consider two locations where the same set of twelves species can be found.
In the first location, the bird community is dominated by just a few species:
In second location, individuals from each species are fairly common:
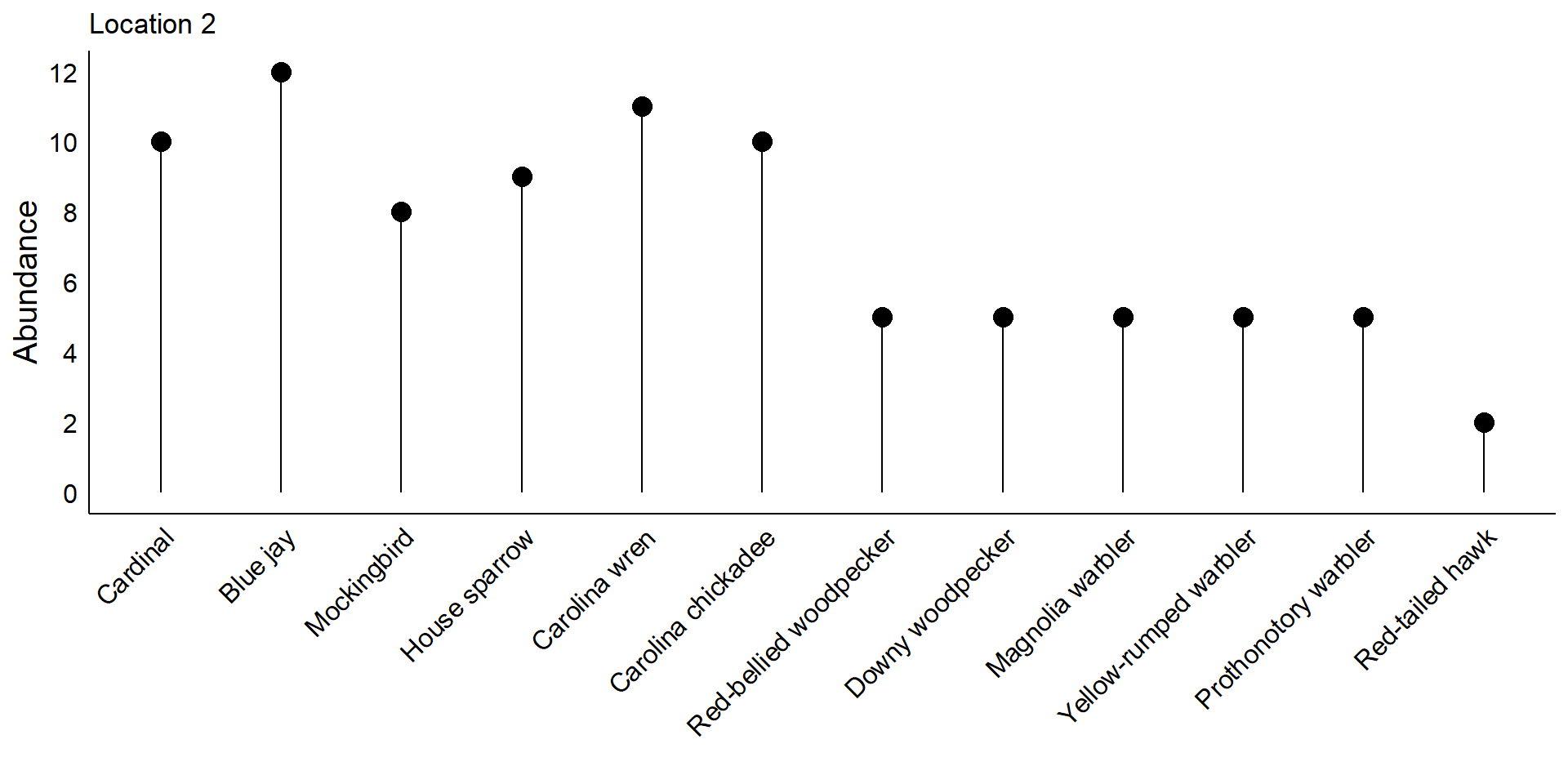
Comparing abundance patterns between the communities
Do the two communities have equal amounts of “biodiversity”?
Quantifying biodiversity
- Ecologists measure biodiversity through a variety of metrics
- One common metric is the Shannon diversity index
Shannon diversity index
Intuition behind the Shannon index
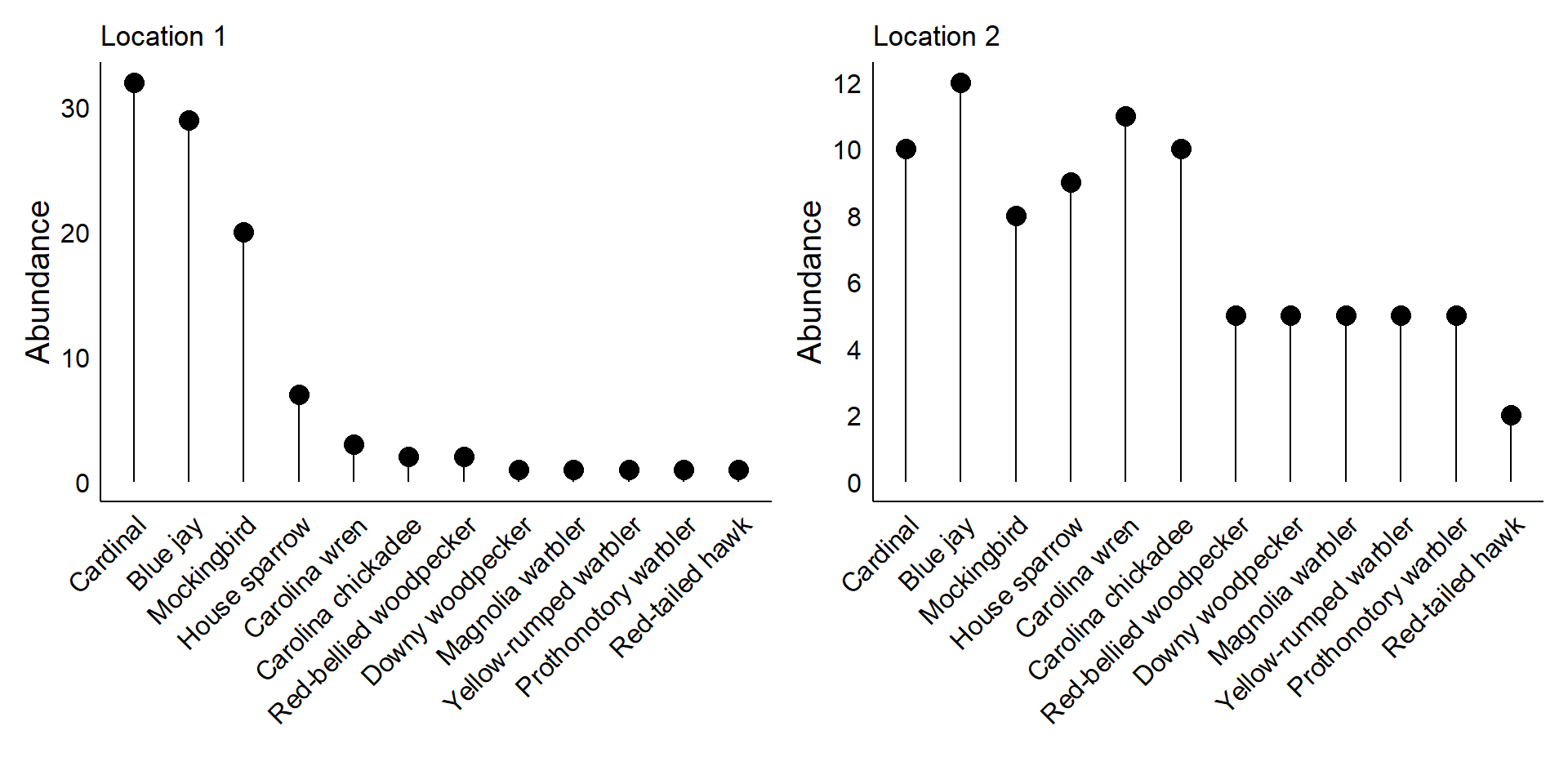
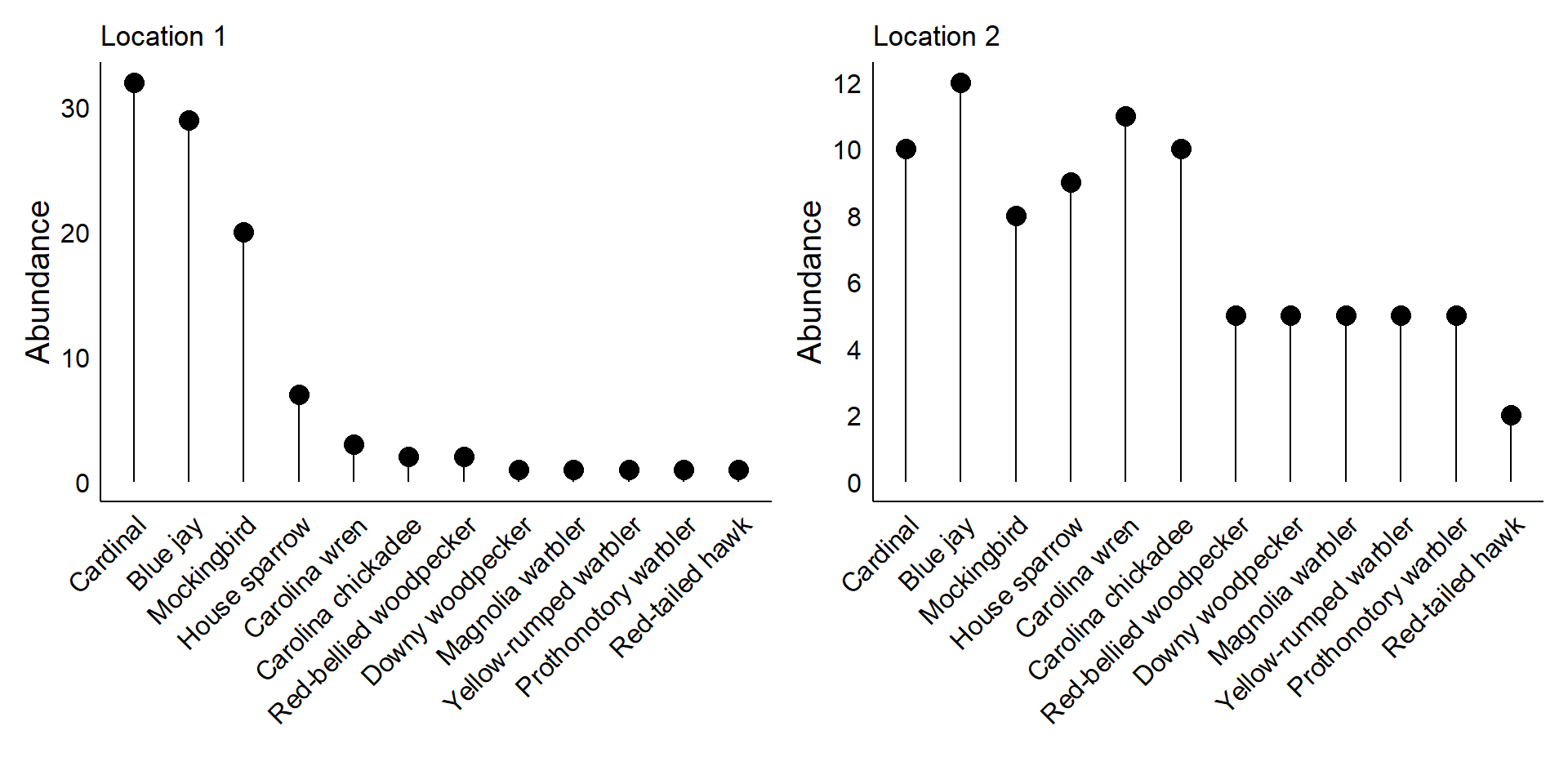
- If I were randomly finding birds in Location 1 and had to guess which species I would find next, which should I guess?
Shannon diversity index
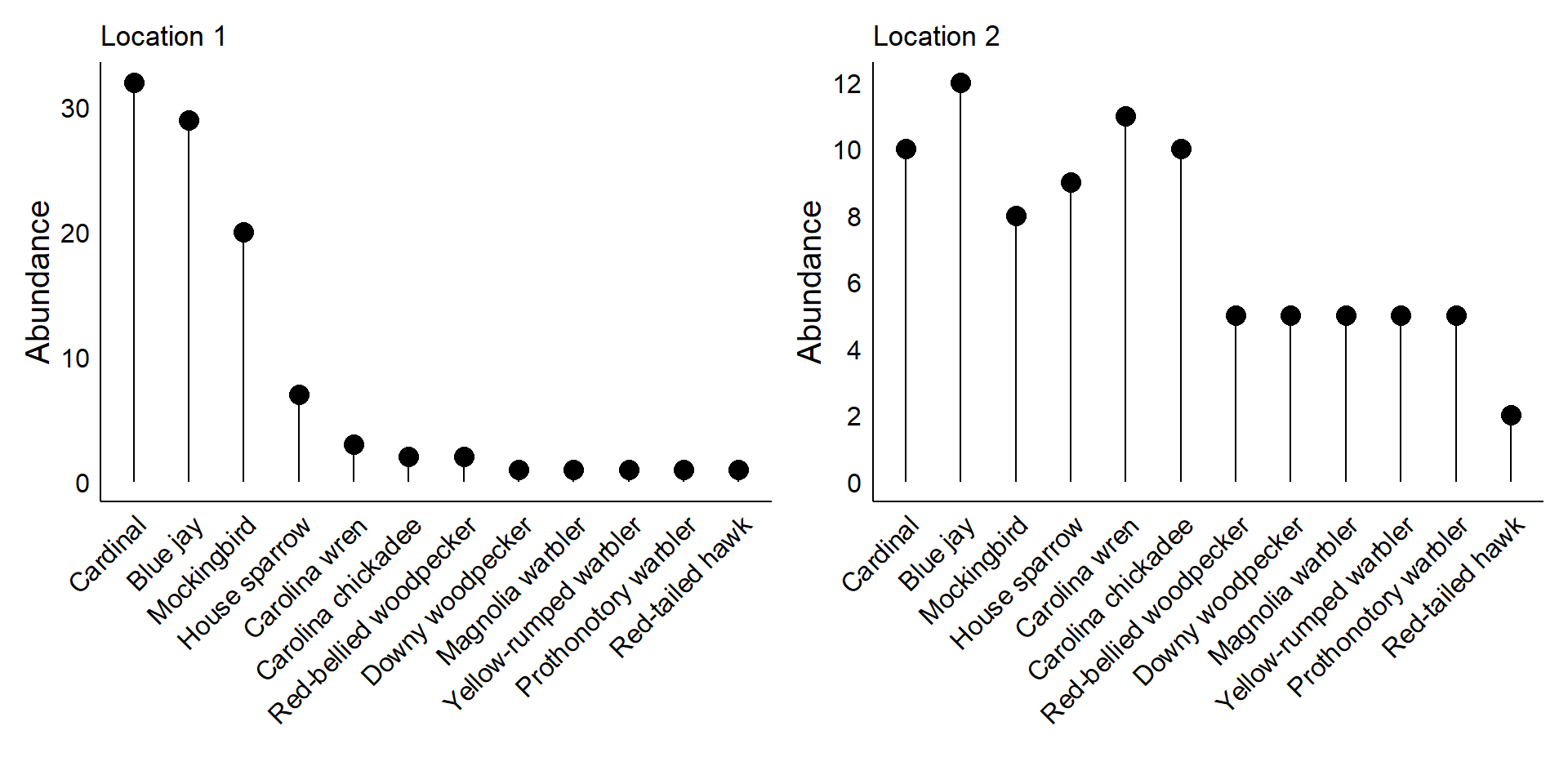
- How about in Location 2?
Shannon diversity index
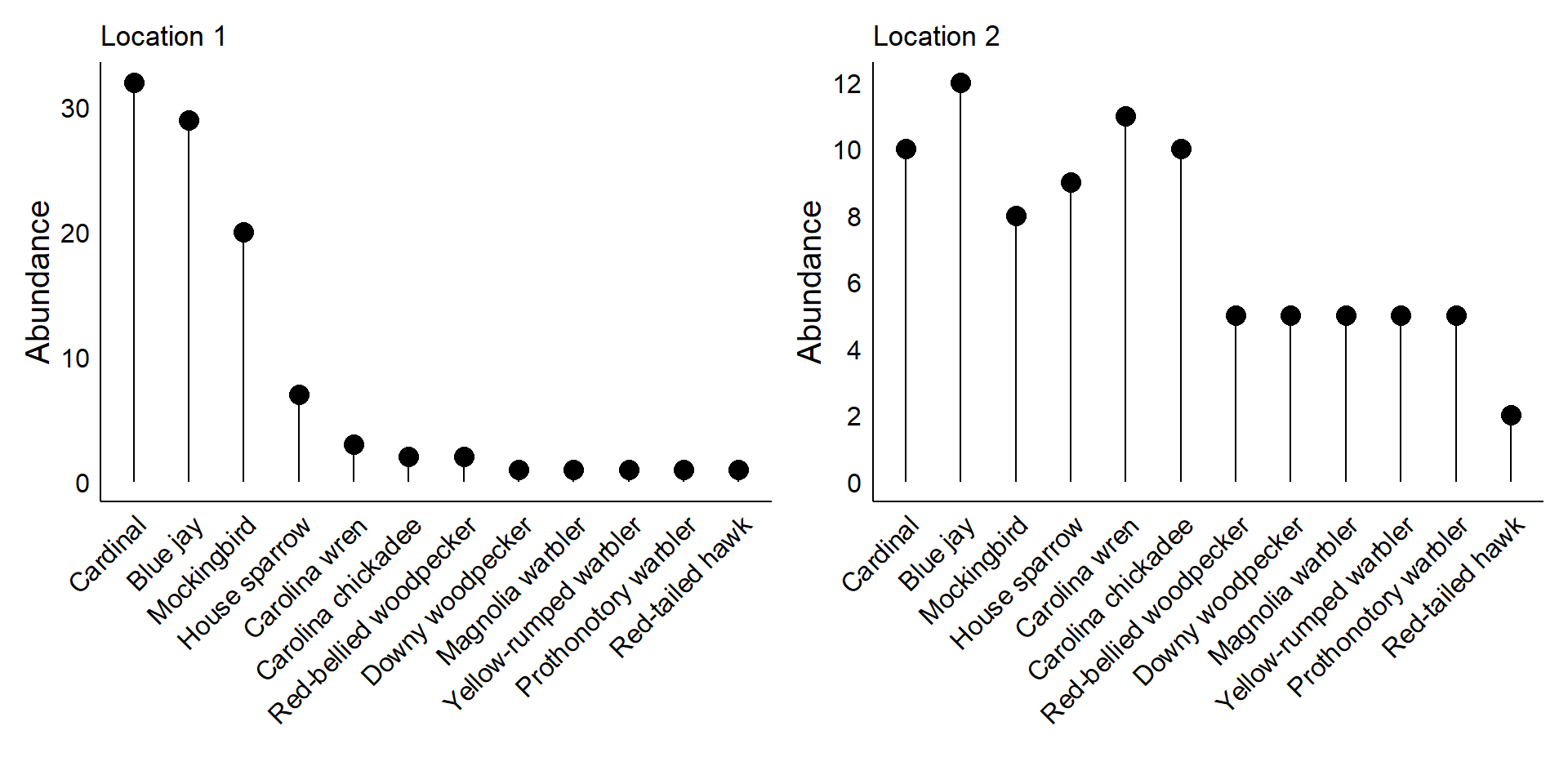
- Location 1 is more “predictable”
- Higher degree of “surprise” in Location 2
- This is also called “Information entropy”
- Commonly used across science, e.g. in computers, to optimize information storage and transfer
Shannon diversity index
A quantitative measure of biodiversity and community “evenness”
Shannonindex⏞H′=−n∑i=1proportionalabundance⏞pi∗log(proportionalabundance)⏞ ln(pi)
Calculating Shannon diversity
| species | Abundance | proportion | log(proportion) | contribution |
|---|---|---|---|---|
| Cardinal | 32 | |||
| Blue jay | 29 | |||
| Mockingbird | 20 | |||
| House sparrow | 7 | |||
| Carolina wren | 3 | |||
| Carolina chickadee | 2 | |||
| Red-bellied woodpecker | 2 | |||
| Downy woodpecker | 1 | |||
| Magnolia warbler | 1 | |||
| Yellow-rumped warbler | 1 | |||
| Prothonotory warbler | 1 | |||
| Red-tailed hawk | 1 |
Calculating Shannon diversity
| species | Abundance | proportion | log(proportion) | contribution |
|---|---|---|---|---|
| Cardinal | 32 | 0.32 | ||
| Blue jay | 29 | 0.29 | ||
| Mockingbird | 20 | 0.20 | ||
| House sparrow | 7 | 0.07 | ||
| Carolina wren | 3 | 0.03 | ||
| Carolina chickadee | 2 | 0.02 | ||
| Red-bellied woodpecker | 2 | 0.02 | ||
| Downy woodpecker | 1 | 0.01 | ||
| Magnolia warbler | 1 | 0.01 | ||
| Yellow-rumped warbler | 1 | 0.01 | ||
| Prothonotory warbler | 1 | 0.01 | ||
| Red-tailed hawk | 1 | 0.01 |
Calculating Shannon diversity
| species | Abundance | proportion | log(proportion) | contribution |
|---|---|---|---|---|
| Cardinal | 32 | 0.32 | -1.139 | |
| Blue jay | 29 | 0.29 | -1.238 | |
| Mockingbird | 20 | 0.20 | -1.609 | |
| House sparrow | 7 | 0.07 | -2.659 | |
| Carolina wren | 3 | 0.03 | -3.507 | |
| Carolina chickadee | 2 | 0.02 | -3.912 | |
| Red-bellied woodpecker | 2 | 0.02 | -3.912 | |
| Downy woodpecker | 1 | 0.01 | -4.605 | |
| Magnolia warbler | 1 | 0.01 | -4.605 | |
| Yellow-rumped warbler | 1 | 0.01 | -4.605 | |
| Prothonotory warbler | 1 | 0.01 | -4.605 | |
| Red-tailed hawk | 1 | 0.01 | -4.605 |
Calculating Shannon diversity
| species | Abundance | proportion | log(proportion) | contribution |
|---|---|---|---|---|
| Cardinal | 32 | 0.32 | -1.139 | -0.364 |
| Blue jay | 29 | 0.29 | -1.238 | -0.359 |
| Mockingbird | 20 | 0.20 | -1.609 | -0.322 |
| House sparrow | 7 | 0.07 | -2.659 | -0.186 |
| Carolina wren | 3 | 0.03 | -3.507 | -0.105 |
| Carolina chickadee | 2 | 0.02 | -3.912 | -0.078 |
| Red-bellied woodpecker | 2 | 0.02 | -3.912 | -0.078 |
| Downy woodpecker | 1 | 0.01 | -4.605 | -0.046 |
| Magnolia warbler | 1 | 0.01 | -4.605 | -0.046 |
| Yellow-rumped warbler | 1 | 0.01 | -4.605 | -0.046 |
| Prothonotory warbler | 1 | 0.01 | -4.605 | -0.046 |
| Red-tailed hawk | 1 | 0.01 | -4.605 | -0.046 |
Exercise: calculating the Shannon index
- Two species lists from eBird, both from BREC Bluebonnet Swamp
- Splitting into groups of 2–3, quantify Shannon diversity
- If time, share something interesting about one of the birds on your list.
Patterns of biodiversity across space
Recap
- At the global scale, the latitudinal diversity gradient is a robust pattern (with exceptions)
- But, quantifying biodiversity isn’t just a matter of counting up species
- Phylogenetic diversity, Functional diversity, Species abundance (and more) dimensions
Recap
If we are sampling individuals from Community 2, there is more uncertainty of which species to expect
We can quantify this uncertainty as the Shannon diversity (H′)
H′=−n∑i=1pi ∗ ln(pi)
Think back: what does this equation mean (in words)?
The geographic scales of biodiversity

pop quiz: how many species of native plants are in Louisiana?
“Did you know that Louisiana has about 2,500 native plants?”
But, not all plants are everywhere!

. . . Bottomland Hardwood Forests and Swamps
Prominent Physical Features: Forested wetlands that occupy broad floodplains and depressions bordering large river systems. The soil, hydrology and plant community vary based on river influence and landscape position. Floodplain soils are fertile and desired for agriculture, so most of the original forests have been converted to agriculture. Flood control efforts have also degraded the forests of this plant region.
Prominent Vegetation: Oaks, cottonwood, sycamores, elms, maples and ashes in bottomland hardwood forests. Bald cypress, water tupelo and swamp tupelo occur in the swamps

Coastal Prairies
Prominent Physical Features: Coastal Prairies Prominent Physical Features: Extension of Midwestern tall-grass prairie, with a subtropical influence. Once covered approximately 2.5 million acres in Louisiana. Modern agriculture has reduced Louisiana’s coastal prairie to less than 1 percent of its former extent. Today, coastal prairie is limited to small remnants on grazing land, along railroads and a few small patches in urban areas. Fire, along with harsh soil conditions, restrict woody species to forests along streams dissecting the plant region
Prominent Vegetation: A diverse mix of lush grasses (little bluestem, big bluestem, eastern gamma grass, switchgrass, and Indian grass), sedges, rushes, and many wildflowers.
There is clearly lots of turnover between regions
Bottomland Hardwood Forests and Swamps
Prominent Vegetation: Oaks, cottonwood, sycamores, elms, maples and ashes in bottomland hardwood forests. Bald cypress, water tupelo and swamp tupelo occur in the swamps
Coastal Prairies
Prominent Vegetation: A diverse mix of lush grasses (little bluestem, big bluestem, eastern gamma grass, switchgrass, and Indian grass), sedges, rushes, and many wildflowers.
Such turnover can also happen on local scales
e.g. Within a forest, the plant community next to a streambed (wet soils) will be different from the plant community at the top of a ridge (dry soils)
Making sense of diversity patterns
The concepts of α, β, and γ diversity help us make sense of these patterns.
- Biodiversity at the largest scale: γ diversity.
- e.g. 2,500 native plants in Louisiana
- Biodiversity at the smallest scale: α diversity.
- e.g. 200 species of grasses and wildflowers at a Coastal Prairie south of Lafayette
- Biodiversity turnover (differences) between local ares: β diversity
- e.g. There are 200 species at Coastal Prairie A and 150 species in Coastal Prairie B, but 50 of the species are unique to Prairie B, and 100 of the species are unique to Prairie A.
Biodiversity patterns in action
In the following communities, each species is represented by a different letter.
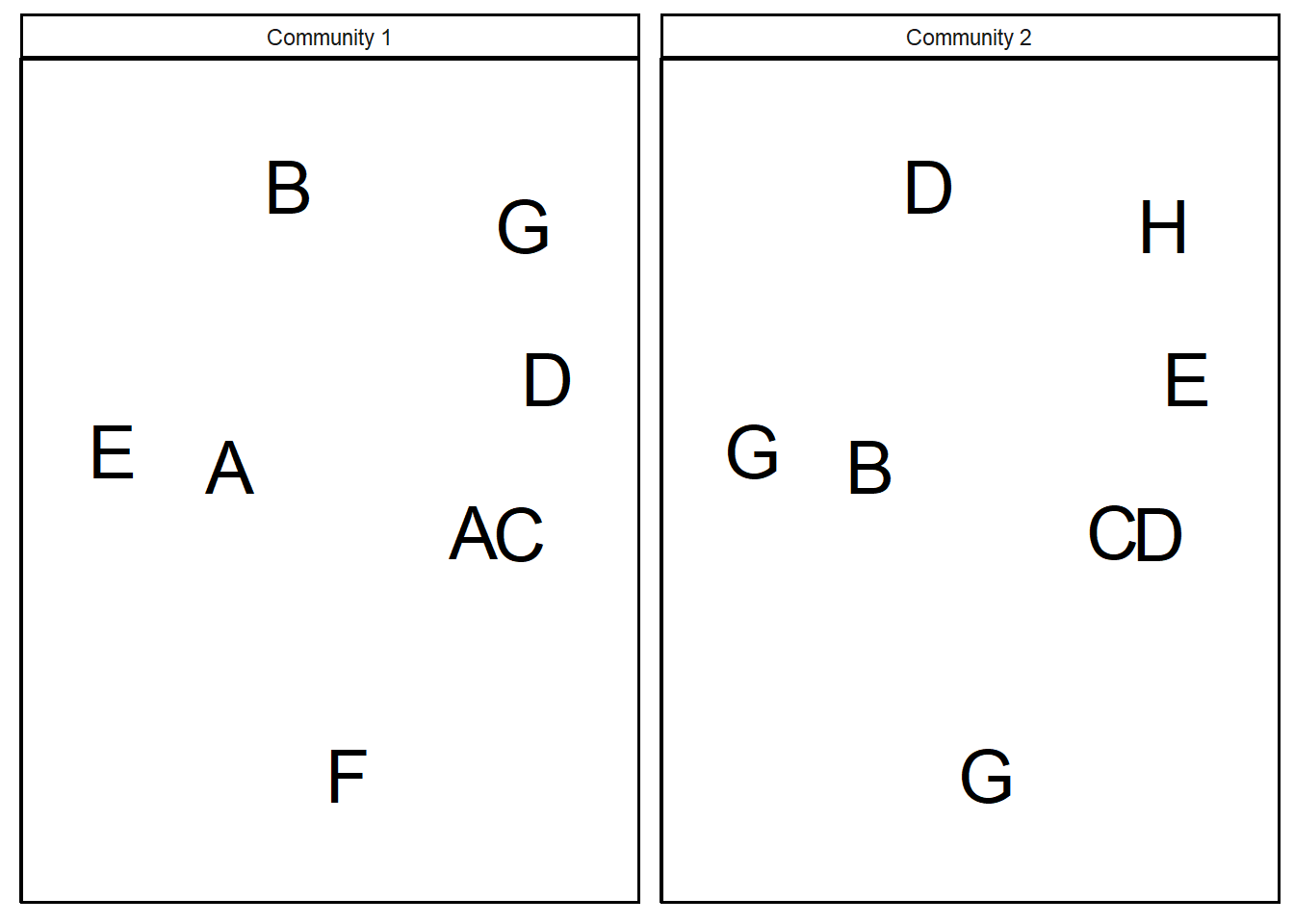
Let’s calculate α, β, and γ diversity.
αcommunity 1=7 species
αcommunity 2=6 species
γ=8 species (A,B,C,D,E,F,G,H)
β is the number of species unique to one community
- Species A and F only in Community 1
- Species H only in Community 2
- β=3 unique species
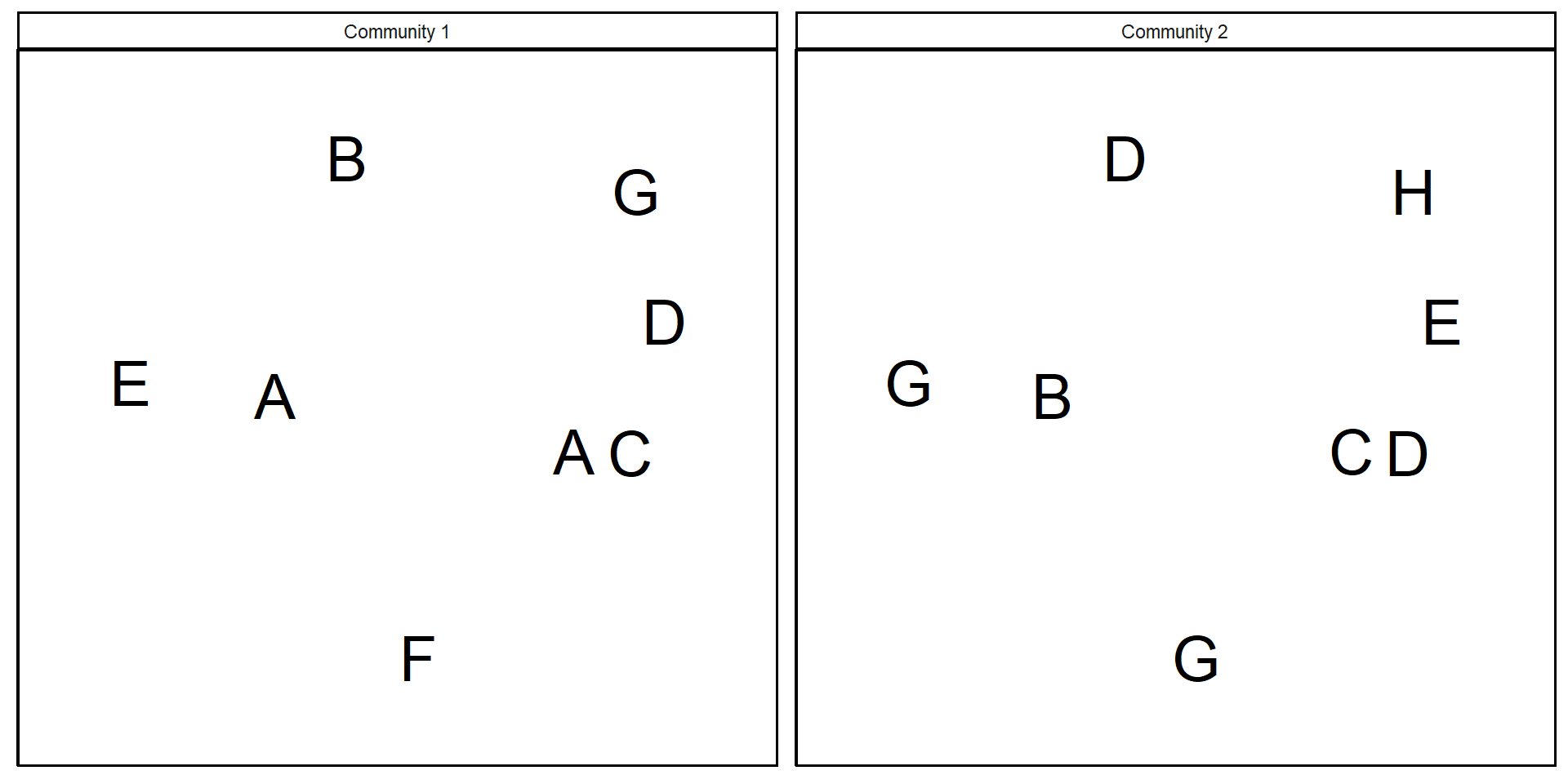
Your turn: calculate α, β, and γ diversity for the following

- β diversity is an important and complicated concept



Why bother?!
- β-diversity is fundamentally an attempt to quantify changes in diversity
- Originally, the concept was developed to understand patterns of species turnover across space (e.g. from the base of a mountain to the top; from the source of a river to its mouth)
- But it has vast implications for some of the most pressing questions today:
- How is biodiversity different at the edge of a forest vs. in its interior?
- How is biodiversity different near cities vs. rural vs. protected areas?
- How is the gut microbiome of someone living in a city different from a person living rurally?
Why bother?!
- β-diversity is fundamentally an attempt to quantify changes in diversity
- Originally, the concept was developed to understand patterns of species turnover across space
- But we can adapt it to ask questions about change in biodiversity over time
- How did biodiversity in the Gulf of Mexico change before/after the oil spill?
- How does the pollinator biodiversity of a neighborhood change when we start planting native flowering plants?
- How does my gut biodiversity change when I change my exercise regime?
Why bother?!
Quantifying patterns of biodiversity is essential for getting a good grasp on managing and protecting biodiversity
More on this in the next two weeks.
Back to understanding patterns of diversity

Why does this pattern exist?
As we often do, let’s simplify the complexity
What about biodiversity patterns at smaller spatial scales?
Start at the simplest level: an isolated area
- i.e. an island that recently formed
- what determines how many species live on it in the long term?
As we often do, let’s simplify the complexity
Processes that govern species richness:
↑ by immigration of new species
↓ by local extinction (‘extirpation’) of existing species
↑ by local speciation
Let’s assume speciation is very slow and not relevant to our dynamics
Processes that govern species richness:
- ↑ by immigration of new species
- ↓ by local extinction (‘extirpation’) of existing species
- What determines rate of immigration of new species and local extinction?
Rates of immigration
What determines rate of immigration?
Definition of immigration: A new species (not already present on the island) arrives for the first time
Number of species already on the island
- If all the species from the mainland are already present on the island, then nothing new can immigrate in
Proximity to source (“mainland”)
- When an island is closer to the source, more new species can end up there

Rates of local extinction
What determines rate of local extinction?
- Number of species already on the island
- If there are lots of species on the island, that means the number of possible extinctions is higher
- Size of island
- On smaller islands, species are more likely to go extinct just by randomness (“stochastic” extinction of smaller populations)

Putting the two together
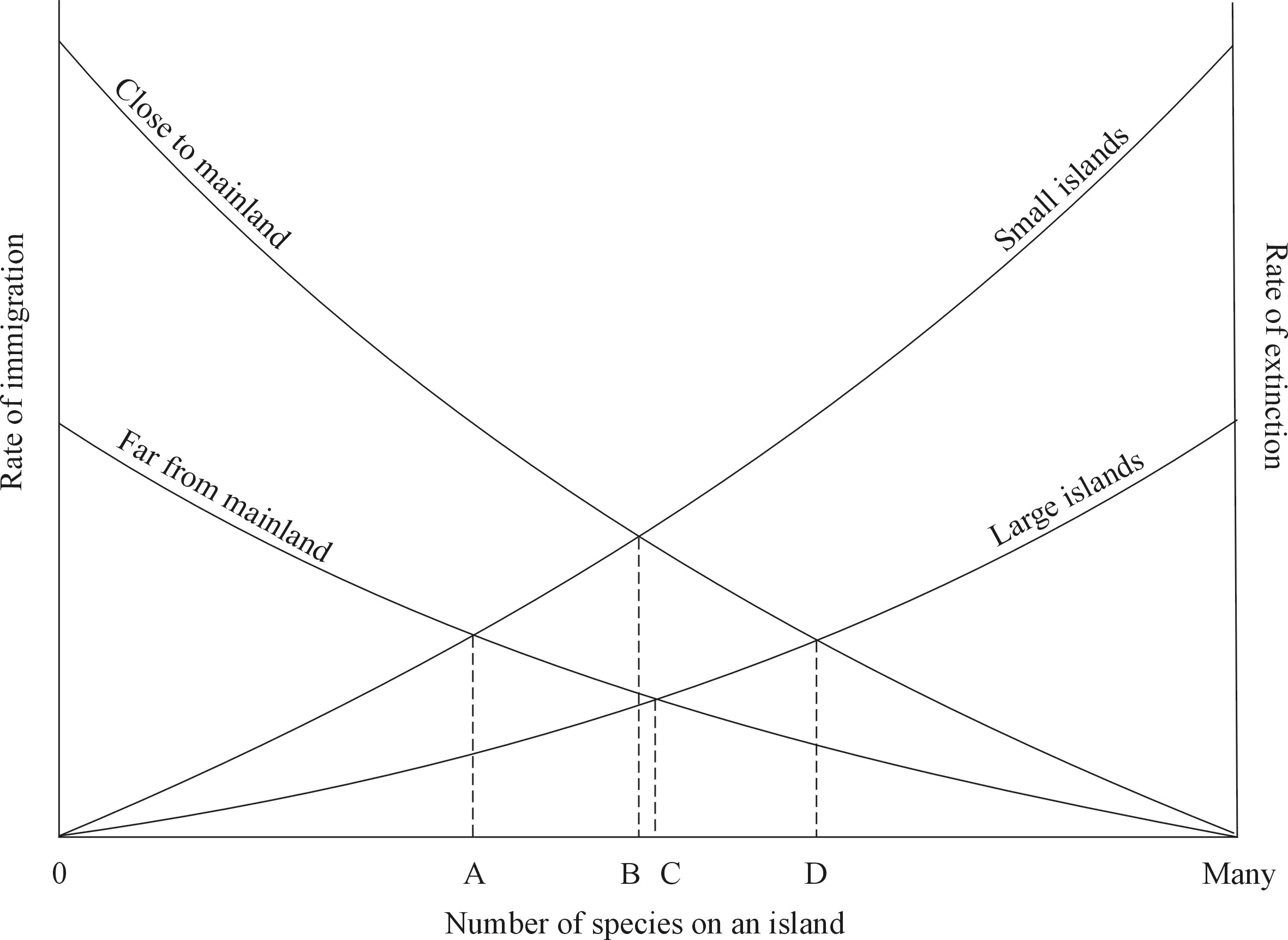
Actual islands are not the only “islands” out there
Urban forests
Lake systems
Application of island biogeography to conservation
